Solar energy [ctd.]
In the previous article, we calculated the luminosity of the Sun and it was found to be about 3.9 1026 W. Later, we realized that our Sun generates energy at a rate equivalent to 2.2×1013 times the global power demand (17.5 TW).
So… One can imagine…
How much energy is dissipated by one square meter on the Sun’s surface?
Let’s find out
Total power output from the Sun= 3.9 1026 W
But radius of the Sun is about 695508 km [1].
So, surface area of the Sun= 4πx (695508 km)2
= 6.08x 1018 m2
Thus, intensity of solar energy at the surface of the Sun=
= 6.41x 107 W m-2
= 64.1 MW m-2
In other words, 64, 100 kJ of energy (64, 100 kW of power) is dissipated by each square meter (per second)!
And, annual energy dissipation by one square meter= 64,100 kW x 365 x 24 h
= 5.62 x 108 kWh
Note that the average electricity consumption per capita in the USA is about 7000 kWh. [2]
So, each square meter (1 m2) of the Sun’s surface dissipates a huge amount of energy equivalent to the cumulative electricity consumption of 80286 Americans.
We can see… how powerful our Sun is.
However, physics of a radiating body does not allow EM waves to travel through space without attenuating with the distance. So, Sun’s radiation starts its journey with an intensity of 6.41x 107 W m-2from the Sun’s surface and diminishes with the distance travels.
As we discussed earlier, the intensity of solar radiation weakens down to 1388 W m-2 (intensity of sunlight on a surface perpendicular to the EM waves) when it reaches the Earth’s atmosphere. In addition to the attenuation of solar energy with distance, it further attenuates owing to the influence of Earth’s atmosphere, as we discussed earlier.
The intensity of solar radiation at a given place on our planet Earth is different from place to place and determined by dozens of factors such as latitude, longitude and weather conditions, etc.
Tropical areas receive a notably higher amount of solar energy than other areas. Obviously, the intensity of sunlight on the Earth’s surface declines with increasing latitude towards the North Pole and decreasing latitude towards the South Pole. Anyway, the intensity of sunlight hitting the Earth’s surface is approximately 1000 W m-2 when the Sun is at the zenith.
Now let us calculate… How much solar power is received by the Earth?
First of all, following facts must be taken into consideration.
- Intensity of sunlight hitting the Earth’s surface when the Sun is at the zenith= 1000 W m-2
- One half of the Earth’s surface is exposed to sunlight at any given point in time.
- Although the intensity of sunlight hitting the Earth’s surface differs from place to place, we can assume that the projected area of the Earth perpendicular to the Sun’s rays is always receiving 1000 W m-2 of power.
Radius of the Earth= 6371 km [3]
Therefore, projected area of the earth perpendicular to the Sun’s rays = π (6371×103 m)2
= 1.27×1014 m2

Image: Projected area of the Earth perpendicular to the Sun’s rays
Solar power received by the Earth = 1.27×1014 m2x1000 W m-2
= 1.27×1012x105 W
= 1.27×105 TW
= 127000 TW
This means our planet receives 127000 TJ of energy, every second.
Note that
The global power demand= 17.5 TW
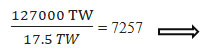 The amount of energy received by the Earth in one day is enough to fulfil the entire global energy demand for almost 7257 days
The amount of energy received by the Earth in one day is enough to fulfil the entire global energy demand for almost 7257 days
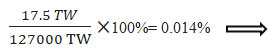 0.014% of the energy received by the Earth is equivalent to the entire global energy demand
0.014% of the energy received by the Earth is equivalent to the entire global energy demand
100%= 0.014%
